Calculating scattering by hand – example 1

Those who read the older SAXS literature will note liberal use of Fourier transforms to calculate the scattering behaviour of odd-shaped particles. Likewise, the effects of smearing due to (for example) beam shape (think “blurring” of the scattering pattern) can be easily determined using such transforms. It is useful to get a feel for the methods for derivation of such Fourier transforms, so I decided it was time to refresh my rusty Fourier transform skills.

[ed: Please remember that the deadline for the SAS2015 conference abstracts is coming up, and that abstracts need to be submitted by the end of this week! The abstract word template is here]

The full derivation is in this document. Here I just highlight the initial and final result, followed by some hints so you can try to figure out the rest as a fun exercise.

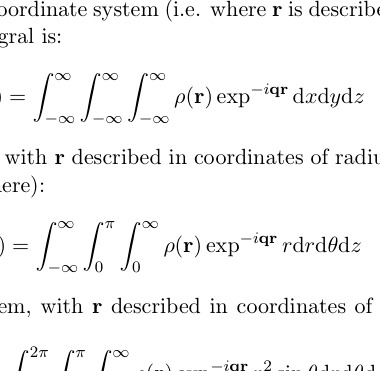
Initial definitions:

We start by defining the Fourier transform itself, which, when applied to scattering, takes the following form [1]:

$F(\mathbf{q}) = \int_v \rho(\mathbf{r}) \exp^{-i\mathbf{qr}} \mathrm{d}v$

For our density function, we can choose a block function, which would be similar to the scattering from square slits (provided the radiation is sufficiently coherent):

$\rho(\mathbf{r}) = \rho(x) \left\{ \begin{array}{lr} \frac{1}{2R} & \textrm{, for } -R \leq x \leq R\\ 0 & \textrm{, for } x < -R, x > R\\ \end{array} \right.$